Deformation Driven Deswelling of Brush Gels
Abstract
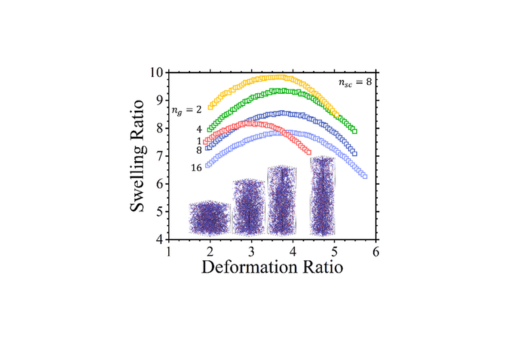
We studied equilibrium swelling in brush gels undergoing large uniaxial deformations in contact with solvent by using a combination of the Flory–Rehner and scaling models of gels and coarse-grained molecular dynamics simulations of swollen brush networks. Our analysis showed that the swelling ratio of the brush gels Qeq(λ), describing the volume change upon swelling, is a nonmonotonic function of the gel uniaxial deformation ratio λ characterizing the gel shape. It first increases with increasing strand elongation from its value for the free-standing gel then begins to decrease. This behavior is a result of the optimization of polymer/solvent interactions and conformational entropy of brush strands in the nonlinear deformation regime. The location of the maximum in the Qeq(λ) function is directly related to the value of the strain-stiffening parameter β. This parameter depends on the degree of polymerization of the brush backbone and the backbone Kuhn length defined by both the grafting density and degree of polymerization of the side chains. Analysis of the nonlinear gel modulus confirmed that the strain-stiffening parameter β is identical in both dry networks and gels. However, the structural modulus of the gels, Gs, is smaller than that of the dry networks, Gdr, by a factor equal to the ratio of the Kuhn length of brush strands in the dry state, bK, and in the gel, bK,s, such that Gs = GdrbK/bK,s.
Citation
Deformation Driven Deswelling of Brush Gels
Michael Jacobs, Yuan Tian, and Andrey V. Dobrynin
Macromolecules 2023 56 (5), 2209-2216
DOI: 10.1021/acs.macromol.2c02385